Skip to content

Latest commit

 

History

History
251 lines (209 loc) · 7.19 KB

README.md

File metadata and controls

251 lines (209 loc) · 7.19 KB
comments difficulty edit_url rating source tags
true
中等
1796
第 379 场周赛 Q2
数学
枚举

3001. 捕获黑皇后需要的最少移动次数

English Version

题目描述

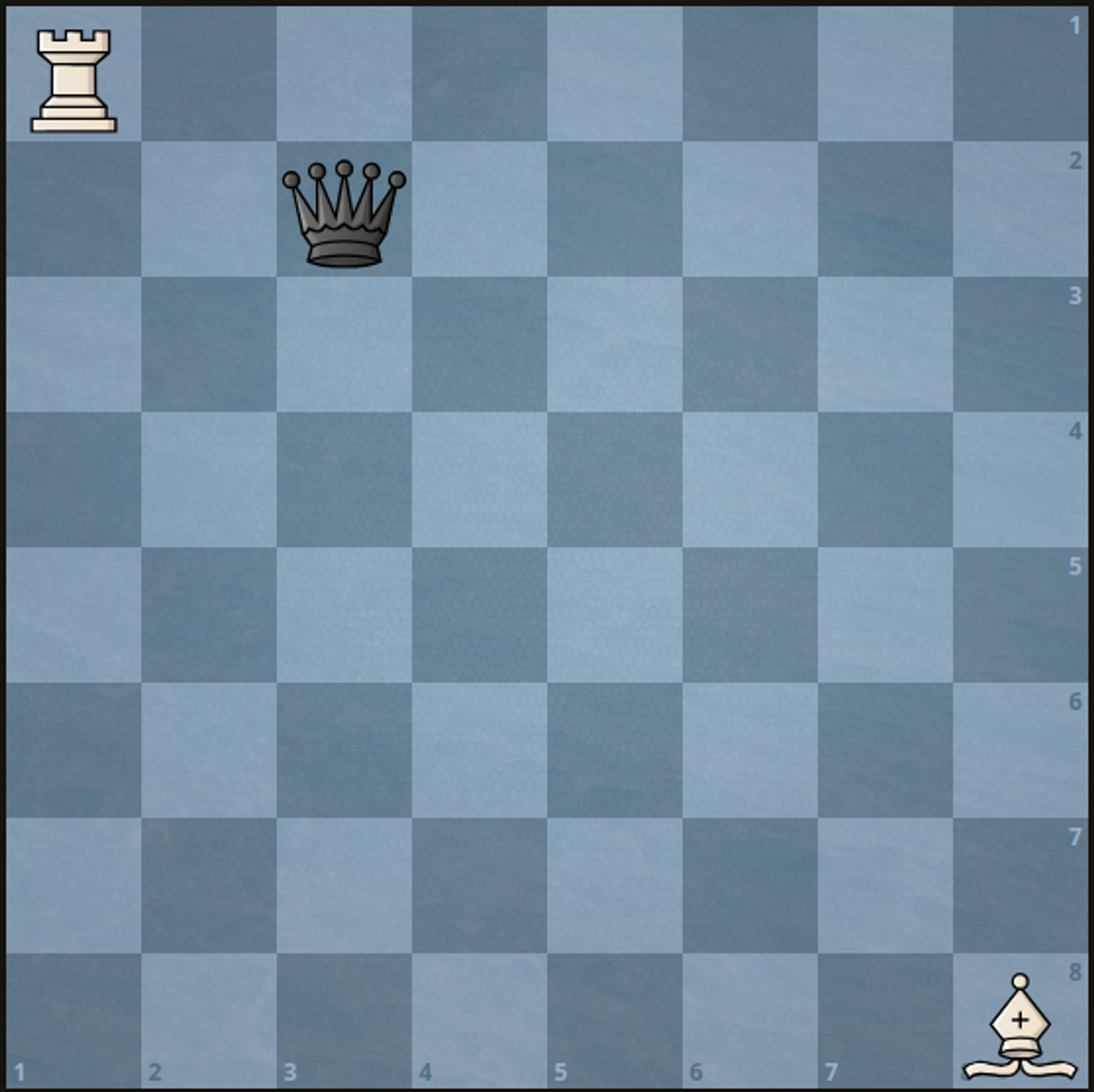
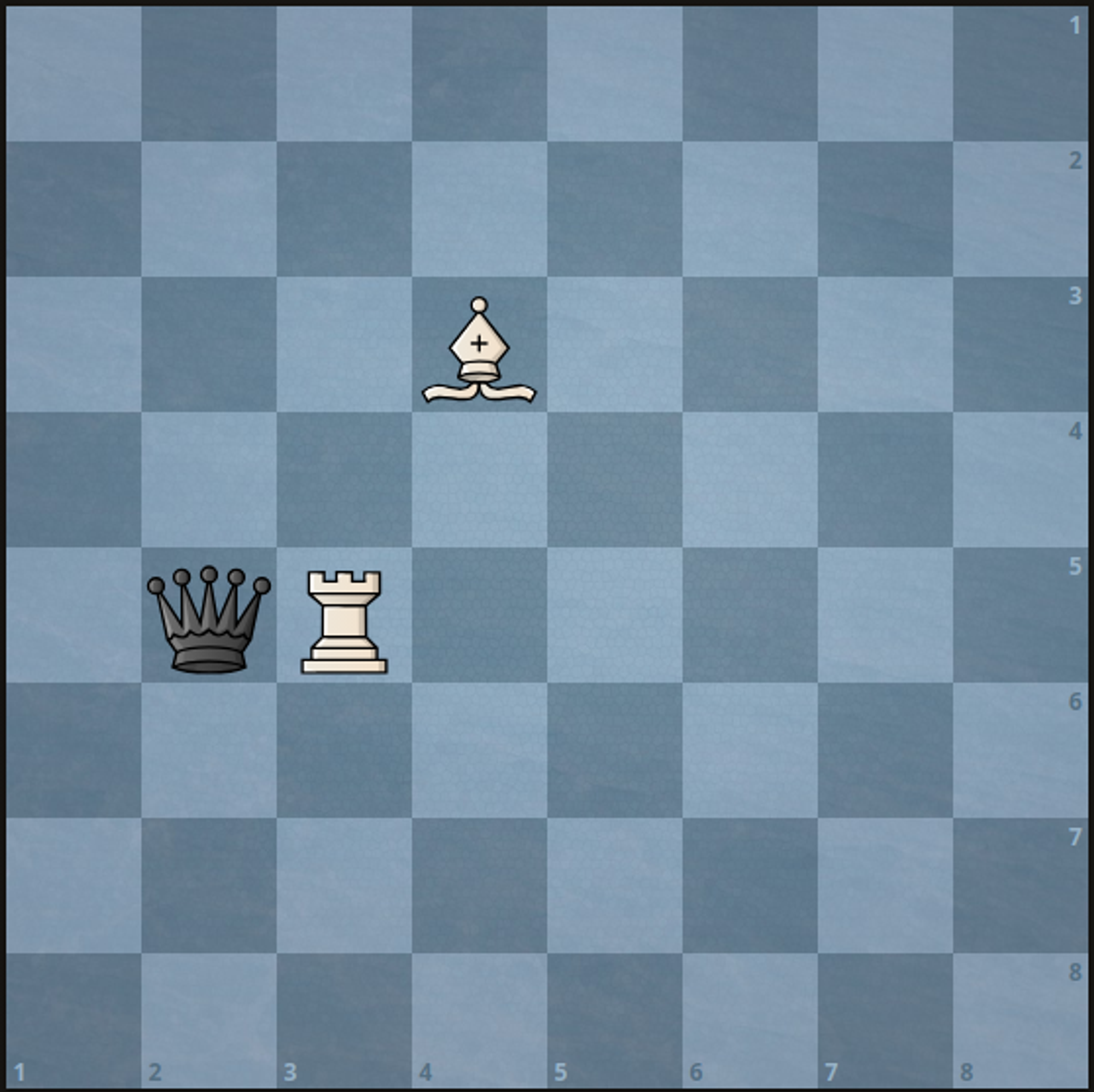
现有一个下标从 1 开始的 8 x 8 棋盘，上面有 3 枚棋子。

给你 6 个整数 a 、b 、c 、d 、e 和 f ，其中：

  • (a, b) 表示白色车的位置。
  • (c, d) 表示白色象的位置。
  • (e, f) 表示黑皇后的位置。

假定你只能移动白色棋子，返回捕获黑皇后所需的最少移动次数。

请注意：

  • 车可以向垂直或水平方向移动任意数量的格子，但不能跳过其他棋子。
  • 象可以沿对角线方向移动任意数量的格子，但不能跳过其他棋子。
  • 如果车或象能移向皇后所在的格子，则认为它们可以捕获皇后。
  • 皇后不能移动。

 

示例 1：

输入：a = 1, b = 1, c = 8, d = 8, e = 2, f = 3
输出：2
解释：将白色车先移动到 (1, 3) ，然后移动到 (2, 3) 来捕获黑皇后，共需移动 2 次。
由于起始时没有任何棋子正在攻击黑皇后，要想捕获黑皇后，移动次数不可能少于 2 次。

示例 2：

输入：a = 5, b = 3, c = 3, d = 4, e = 5, f = 2
输出：1
解释：可以通过以下任一方式移动 1 次捕获黑皇后：
- 将白色车移动到 (5, 2) 。
- 将白色象移动到 (5, 2) 。

 

提示：

  • 1 <= a, b, c, d, e, f <= 8
  • 两枚棋子不会同时出现在同一个格子上。

解法

方法一：分类讨论

根据题意，我们可以将捕获黑皇后的情况分为以下几种：

  1. 白色车和黑皇后在同一行，且中间没有其他棋子，此时只需要移动白色车 $1$ 一次；
  2. 白色车和黑皇后在同一列，且中间没有其他棋子，此时只需要移动白色车 $1$ 一次；
  3. 白色象和黑皇后在对角线 \ 上，且中间没有其他棋子，此时只需要移动白色象 $1$ 一次；
  4. 白色象和黑皇后在对角线 / 上，且中间没有其他棋子，此时只需要移动白色象 $1$ 一次；
  5. 其他情况，只需要移动两次。

时间复杂度 $O(1)$，空间复杂度 $O(1)$。

Python3

class Solution:
    def minMovesToCaptureTheQueen(
        self, a: int, b: int, c: int, d: int, e: int, f: int
    ) -> int:
        if a == e and (c != a or (d - b) * (d - f) > 0):
            return 1
        if b == f and (d != b or (c - a) * (c - e) > 0):
            return 1
        if c - e == d - f and (a - e != b - f or (a - c) * (a - e) > 0):
            return 1
        if c - e == f - d and (a - e != f - b or (a - c) * (a - e) > 0):
            return 1
        return 2

Java

class Solution {
    public int minMovesToCaptureTheQueen(int a, int b, int c, int d, int e, int f) {
        if (a == e && (c != a || (d - b) * (d - f) > 0)) {
            return 1;
        }
        if (b == f && (d != b || (c - a) * (c - e) > 0)) {
            return 1;
        }
        if (c - e == d - f && (a - e != b - f || (a - c) * (a - e) > 0)) {
            return 1;
        }
        if (c - e == f - d && (a - e != f - b || (a - c) * (a - e) > 0)) {
            return 1;
        }
        return 2;
    }
}

C++

class Solution {
public:
    int minMovesToCaptureTheQueen(int a, int b, int c, int d, int e, int f) {
        if (a == e && (c != a || (d - b) * (d - f) > 0)) {
            return 1;
        }
        if (b == f && (d != b || (c - a) * (c - e) > 0)) {
            return 1;
        }
        if (c - e == d - f && (a - e != b - f || (a - c) * (a - e) > 0)) {
            return 1;
        }
        if (c - e == f - d && (a - e != f - b || (a - c) * (a - e) > 0)) {
            return 1;
        }
        return 2;
    }
};

Go

func minMovesToCaptureTheQueen(a int, b int, c int, d int, e int, f int) int {
	if a == e && (c != a || (d-b)*(d-f) > 0) {
		return 1
	}
	if b == f && (d != b || (c-a)*(c-e) > 0) {
		return 1
	}
	if c-e == d-f && (a-e != b-f || (a-c)*(a-e) > 0) {
		return 1
	}
	if c-e == f-d && (a-e != f-b || (a-c)*(a-e) > 0) {
		return 1
	}
	return 2
}

TypeScript

function minMovesToCaptureTheQueen(
    a: number,
    b: number,
    c: number,
    d: number,
    e: number,
    f: number,
): number {
    if (a === e && (c !== a || (d - b) * (d - f) > 0)) {
        return 1;
    }
    if (b === f && (d !== b || (c - a) * (c - e) > 0)) {
        return 1;
    }
    if (c - e === d - f && (a - e !== b - f || (a - c) * (a - e) > 0)) {
        return 1;
    }
    if (c - e === f - d && (a - e !== f - b || (a - c) * (a - e) > 0)) {
        return 1;
    }
    return 2;
}

Rust

impl Solution {
    pub fn min_moves_to_capture_the_queen(a: i32, b: i32, c: i32, d: i32, e: i32, f: i32) -> i32 {
        if a == e && (c != a || (d - b) * (d - f) > 0) {
            return 1;
        }
        if b == f && (d != b || (c - a) * (c - e) > 0) {
            return 1;
        }
        if c - e == d - f && (a - e != b - f || (a - c) * (a - e) > 0) {
            return 1;
        }
        if c - e == f - d && (a - e != f - b || (a - c) * (a - e) > 0) {
            return 1;
        }
        return 2;
    }
}

Cangjie

class Solution {
    func minMovesToCaptureTheQueen(a: Int64, b: Int64, c: Int64, d: Int64, e: Int64, f: Int64): Int64 {
        if (a == e && (c != a || (d - b) * (d - f) > 0)) {
            return 1
        }
        if (b == f && (d != b || (c - a) * (c - e) > 0)) {
            return 1
        }
        if (c - e == d - f && (a - e != b - f || (a - c) * (a - e) > 0)) {
            return 1
        }
        if (c - e == f - d && (a - e != f - b || (a - c) * (a - e) > 0)) {
            return 1
        }
        2
    }
}