Skip to content

Latest commit

 

History

History
273 lines (225 loc) · 7.88 KB

README.md

File metadata and controls

273 lines (225 loc) · 7.88 KB
comments difficulty edit_url tags
true
中等
树
深度优先搜索
广度优先搜索
哈希表
二叉树

865. 具有所有最深节点的最小子树

English Version

题目描述

给定一个根为 root 的二叉树，每个节点的深度是 该节点到根的最短距离 。

返回包含原始树中所有 最深节点 的 最小子树 。

如果一个节点在 整个树 的任意节点之间具有最大的深度，则该节点是 最深的 。

一个节点的 子树 是该节点加上它的所有后代的集合。

 

示例 1：

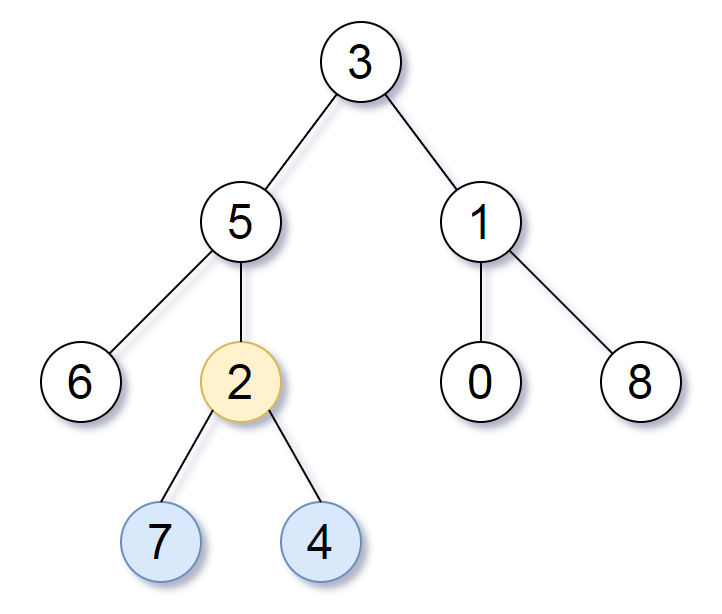
输入：root = [3,5,1,6,2,0,8,null,null,7,4]
输出：[2,7,4]
解释：
我们返回值为 2 的节点，在图中用黄色标记。
在图中用蓝色标记的是树的最深的节点。
注意，节点 5、3 和 2 包含树中最深的节点，但节点 2 的子树最小，因此我们返回它。

示例 2：

输入：root = [1]
输出：[1]
解释：根节点是树中最深的节点。

示例 3：

输入：root = [0,1,3,null,2]
输出：[2]
解释：树中最深的节点为 2 ，有效子树为节点 2、1 和 0 的子树，但节点 2 的子树最小。

 

提示：

  • 树中节点的数量在 [1, 500] 范围内。
  • 0 <= Node.val <= 500
  • 每个节点的值都是 独一无二 的。

 

注意：本题与力扣 1123 重复：https://leetcode.cn/problems/lowest-common-ancestor-of-deepest-leaves

解法

方法一：递归

我们设计一个函数 $\textit{dfs}(\textit{root})$，返回以 $\textit{root}$ 为根的子树中，包含所有最深节点的最小子树，以及以 $\textit{root}$ 为根的子树的深度。

函数 $\textit{dfs}(\textit{root})$ 的执行过程如下：

  • 如果 $\textit{root}$ 为空，返回 $\text{null}$ 和 $0$。
  • 否则，递归计算 $\textit{root}$ 的左子树和右子树的最小子树以及深度，分别为 $l$ 和 $l_d$ 以及 $r$ 和 $r_d$。如果 $l_d &gt; r_d$，则以 $\textit{root}$ 的左孩子为根的子树中包含所有最深节点的最小子树就是 $l$，深度为 $l_d + 1$；如果 $l_d &lt; r_d$，则以 $\textit{root}$ 的右孩子为根的子树中包含所有最深节点的最小子树就是 $r$，深度为 $r_d + 1$；如果 $l_d = r_d$，则 $\textit{root}$ 就是包含所有最深节点的最小子树，深度为 $l_d + 1$。

最后，返回 $\textit{dfs}(\textit{root})$ 的结果的第一个元素即可。

时间复杂度 $O(n)$，空间复杂度 $O(n)$。其中 $n$ 为二叉树的节点个数。

Python3

# Definition for a binary tree node.
# class TreeNode:
#     def __init__(self, val=0, left=None, right=None):
#         self.val = val
#         self.left = left
#         self.right = right
class Solution:
    def subtreeWithAllDeepest(self, root: Optional[TreeNode]) -> Optional[TreeNode]:
        def dfs(root: Optional[TreeNode]) -> Tuple[Optional[TreeNode], int]:
            if root is None:
                return None, 0
            l, ld = dfs(root.left)
            r, rd = dfs(root.right)
            if ld > rd:
                return l, ld + 1
            if ld < rd:
                return r, rd + 1
            return root, ld + 1

        return dfs(root)[0]

Java

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     int val;
 *     TreeNode left;
 *     TreeNode right;
 *     TreeNode() {}
 *     TreeNode(int val) { this.val = val; }
 *     TreeNode(int val, TreeNode left, TreeNode right) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
class Solution {
    public TreeNode subtreeWithAllDeepest(TreeNode root) {
        return dfs(root).getKey();
    }

    private Pair<TreeNode, Integer> dfs(TreeNode root) {
        if (root == null) {
            return new Pair<>(null, 0);
        }
        var l = dfs(root.left);
        var r = dfs(root.right);
        int ld = l.getValue(), rd = r.getValue();
        if (ld > rd) {
            return new Pair<>(l.getKey(), ld + 1);
        }
        if (ld < rd) {
            return new Pair<>(r.getKey(), rd + 1);
        }
        return new Pair<>(root, ld + 1);
    }
}

C++

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    TreeNode* subtreeWithAllDeepest(TreeNode* root) {
        using pti = pair<TreeNode*, int>;
        auto dfs = [&](this auto&& dfs, TreeNode* root) -> pti {
            if (!root) {
                return {nullptr, 0};
            }
            auto [l, ld] = dfs(root->left);
            auto [r, rd] = dfs(root->right);
            if (ld > rd) {
                return {l, ld + 1};
            }
            if (ld < rd) {
                return {r, rd + 1};
            }
            return {root, ld + 1};
        };
        return dfs(root).first;
    }
};

Go

/**
 * Definition for a binary tree node.
 * type TreeNode struct {
 *     Val int
 *     Left *TreeNode
 *     Right *TreeNode
 * }
 */
func subtreeWithAllDeepest(root *TreeNode) *TreeNode {
	type pair struct {
		node  *TreeNode
		depth int
	}
	var dfs func(*TreeNode) pair
	dfs = func(root *TreeNode) pair {
		if root == nil {
			return pair{nil, 0}
		}
		l, r := dfs(root.Left), dfs(root.Right)
		ld, rd := l.depth, r.depth
		if ld > rd {
			return pair{l.node, ld + 1}
		}
		if ld < rd {
			return pair{r.node, rd + 1}
		}
		return pair{root, ld + 1}
	}
	return dfs(root).node
}

TypeScript

/**
 * Definition for a binary tree node.
 * class TreeNode {
 *     val: number
 *     left: TreeNode | null
 *     right: TreeNode | null
 *     constructor(val?: number, left?: TreeNode | null, right?: TreeNode | null) {
 *         this.val = (val===undefined ? 0 : val)
 *         this.left = (left===undefined ? null : left)
 *         this.right = (right===undefined ? null : right)
 *     }
 * }
 */

function subtreeWithAllDeepest(root: TreeNode | null): TreeNode | null {
    const dfs = (root: TreeNode | null): [TreeNode, number] => {
        if (!root) {
            return [null, 0];
        }
        const [l, ld] = dfs(root.left);
        const [r, rd] = dfs(root.right);
        if (ld > rd) {
            return [l, ld + 1];
        }
        if (ld < rd) {
            return [r, rd + 1];
        }
        return [root, ld + 1];
    };
    return dfs(root)[0];
}