README.md

comments	difficulty	edit_url	tags
true	简单	https://github.com/doocs/leetcode/edit/main/solution/0200-0299/0226.Invert%20Binary%20Tree/README.md	树 深度优先搜索 广度优先搜索 二叉树

226. 翻转二叉树

English Version

题目描述

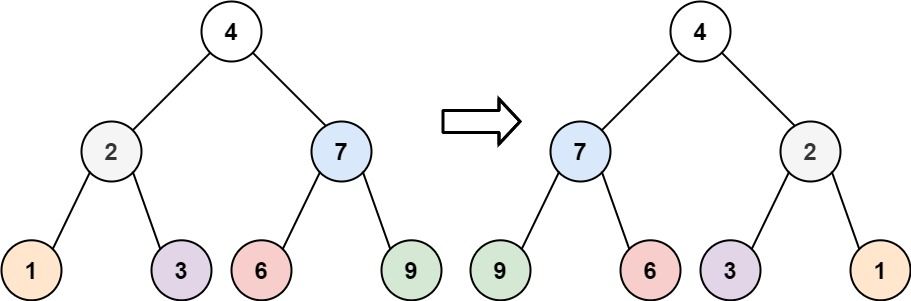
给你一棵二叉树的根节点 root ，翻转这棵二叉树，并返回其根节点。

 

示例 1：

输入：root = [4,2,7,1,3,6,9]
输出：[4,7,2,9,6,3,1]

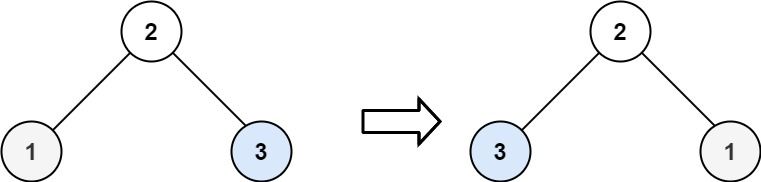
示例 2：

输入：root = [2,1,3]
输出：[2,3,1]

示例 3：

输入：root = []
输出：[]

 

提示：

• 树中节点数目范围在 [0, 100] 内
• -100 <= Node.val <= 100

解法

方法一：递归

我们首先判断 $\textit{root}$ 是否为空，若为空则直接返回 $\text{null}$。然后递归地对树的左右子树进行翻转，将翻转后的右子树作为新的左子树，将翻转后的左子树作为新的右子树，返回 $\textit{root}$。

时间复杂度 $O(n)$，空间复杂度 $O(n)$。其中 $n$ 是二叉树的节点个数。

Python3

# Definition for a binary tree node.
# class TreeNode:
#     def __init__(self, val=0, left=None, right=None):
#         self.val = val
#         self.left = left
#         self.right = right
class Solution:
    def invertTree(self, root: Optional[TreeNode]) -> Optional[TreeNode]:
        if root is None:
            return None
        l, r = self.invertTree(root.left), self.invertTree(root.right)
        root.left, root.right = r, l
        return root

Java

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     int val;
 *     TreeNode left;
 *     TreeNode right;
 *     TreeNode() {}
 *     TreeNode(int val) { this.val = val; }
 *     TreeNode(int val, TreeNode left, TreeNode right) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
class Solution {
    public TreeNode invertTree(TreeNode root) {
        if (root == null) {
            return null;
        }
        TreeNode l = invertTree(root.left);
        TreeNode r = invertTree(root.right);
        root.left = r;
        root.right = l;
        return root;
    }
}

C++

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    TreeNode* invertTree(TreeNode* root) {
        if (!root) {
            return root;
        }
        TreeNode* l = invertTree(root->left);
        TreeNode* r = invertTree(root->right);
        root->left = r;
        root->right = l;
        return root;
    }
};

Go

/**
 * Definition for a binary tree node.
 * type TreeNode struct {
 *     Val int
 *     Left *TreeNode
 *     Right *TreeNode
 * }
 */
func invertTree(root *TreeNode) *TreeNode {
	if root == nil {
		return root
	}
	l, r := invertTree(root.Left), invertTree(root.Right)
	root.Left, root.Right = r, l
	return root
}

TypeScript

/**
 * Definition for a binary tree node.
 * class TreeNode {
 *     val: number
 *     left: TreeNode | null
 *     right: TreeNode | null
 *     constructor(val?: number, left?: TreeNode | null, right?: TreeNode | null) {
 *         this.val = (val===undefined ? 0 : val)
 *         this.left = (left===undefined ? null : left)
 *         this.right = (right===undefined ? null : right)
 *     }
 * }
 */

function invertTree(root: TreeNode | null): TreeNode | null {
    if (!root) {
        return root;
    }
    const l = invertTree(root.left);
    const r = invertTree(root.right);
    root.left = r;
    root.right = l;
    return root;
}

Rust

// Definition for a binary tree node.
// #[derive(Debug, PartialEq, Eq)]
// pub struct TreeNode {
//   pub val: i32,
//   pub left: Option<Rc<RefCell<TreeNode>>>,
//   pub right: Option<Rc<RefCell<TreeNode>>>,
// }
//
// impl TreeNode {
//   #[inline]
//   pub fn new(val: i32) -> Self {
//     TreeNode {
//       val,
//       left: None,
//       right: None
//     }
//   }
// }
use std::rc::Rc;
use std::cell::RefCell;
impl Solution {
    pub fn invert_tree(root: Option<Rc<RefCell<TreeNode>>>) -> Option<Rc<RefCell<TreeNode>>> {
        if let Some(node) = root.clone() {
            let mut node = node.borrow_mut();
            let left = node.left.take();
            let right = node.right.take();
            node.left = Self::invert_tree(right);
            node.right = Self::invert_tree(left);
        }
        root
    }
}

JavaScript

/**
 * Definition for a binary tree node.
 * function TreeNode(val, left, right) {
 *     this.val = (val===undefined ? 0 : val)
 *     this.left = (left===undefined ? null : left)
 *     this.right = (right===undefined ? null : right)
 * }
 */
/**
 * @param {TreeNode} root
 * @return {TreeNode}
 */
var invertTree = function (root) {
    if (!root) {
        return root;
    }
    const l = invertTree(root.left);
    const r = invertTree(root.right);
    root.left = r;
    root.right = l;
    return root;
};

C#

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    public TreeNode InvertTree(TreeNode root) {
        if (root == null) {
            return null;
        }
        TreeNode l = InvertTree(root.left);
        TreeNode r = InvertTree(root.right);
        root.left = r;
        root.right = l;
        return root;
    }
}